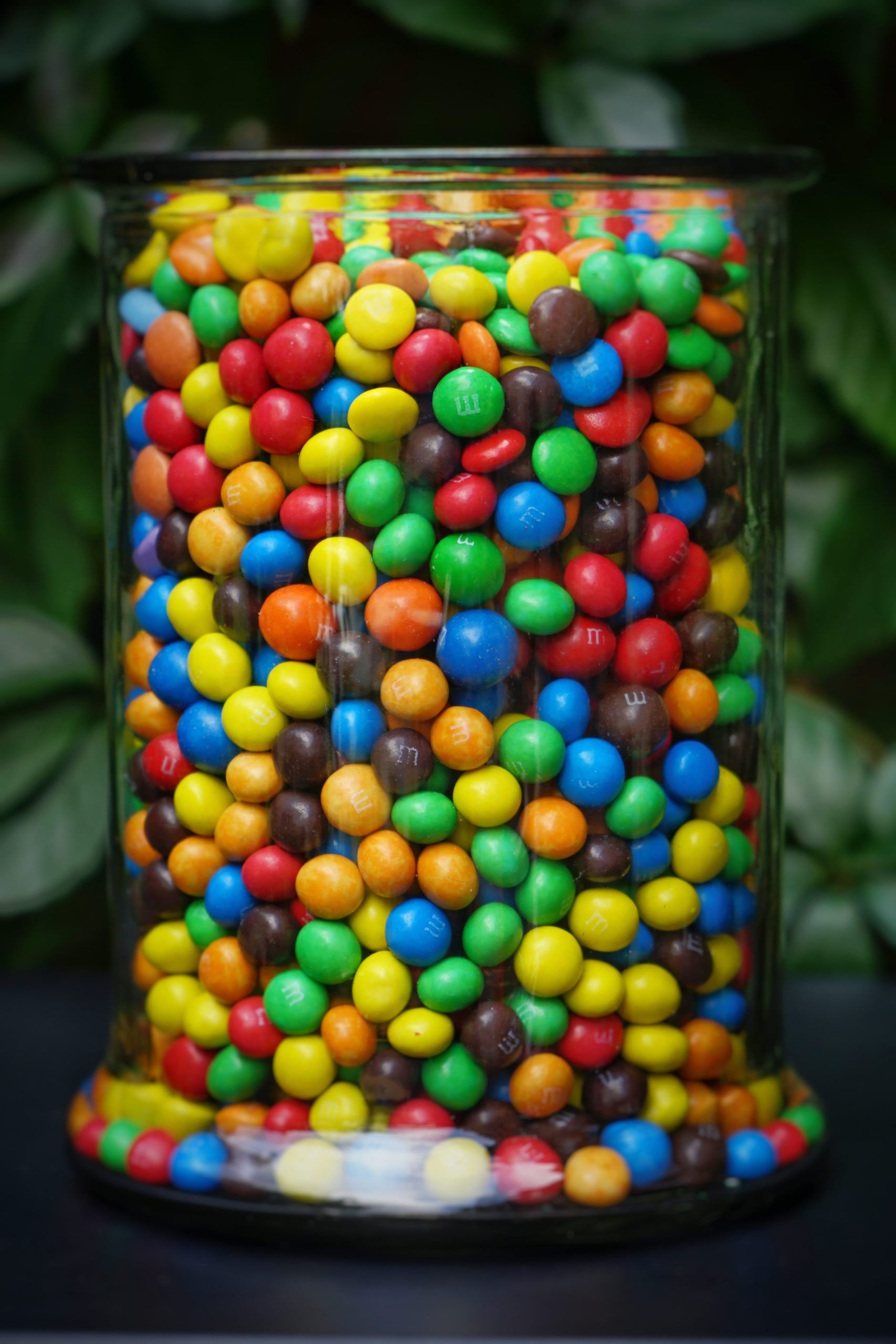Author: Cvetanka Trifunovska, Quality Assurance
Here is my explanation for sweet M&M Challenge for winning tickets for Voxxed Days in Belgrade 2016.
The problem
The Solution
There are 2 lemonade jars. They look like quart jars (capacity: 946 ml= 946 cm³).
Firstly, i will calculate the number in the jar, that has only regular M&M…
Regular M&M bonbons has diameter around d=1,356 cm and volume around 0,636 cm³. So, according to the video, i will count the bonbons, to calculate the radius and high of the jar. (and i done a little research about radius of a jar, it’s around 8,6 cm). At the video: d= 5 to 6 M&M bonbons in a line, so i would say that d = 8 cm => r = 4 cm. High is around 4 M&Ms, h=5,242.
So, V regular = pi ⋅ r² ⋅ h = 3,14 ⋅ 16 ⋅ 5,242 = 272,5 cm³
But, not the all volume is filled with M&Ms. Around 68% of the jar is filled with M&Ms and the other part is air.
Vregular = 272,5 ⋅ 68/100 = 180,3 cm³
nr = 180,3 : 0,636 ≃ 291 bonbons
Let’s calculate how many bonbons are in full jar with regular and peanut M&Ms. Peanut M&Ms are not remarkably bigger than regular M&Ms from (2 -3 cm³), so i would say around 2,5 cm³. Volume of the all peanuts is 80% from the volume of the jar, since peanuts M&M are filling much more space that the regular M&M.
In best case:
Vregular + Vpeanut = 2,5 + 0,636 = 3,136 cm³
nmix= 946 : 3,136 ≃ 301
80% out of 301 is 301 ⋅ 80 : 100 = 240 bonbons
68% out of 301 is 301 ⋅ 68 : 100 = 205 bonbons
So difference between those is 10, 61, 96.
Case when jars will have the most difference in bonbons:
nreg = 946 : 0,636 = 1487, 68% from nreg = 1011 bonbons
npean = 946 : 2,5 = 378, 80% from npean = 302 bonbons
90% of 1011 and 10% of 302 = 909 + 30 = 930
Difference is 930 – 291 = 639
Case when jars will have small difference in bonbons:
90% of 302 +10% of 1011 = 271 + 101 = 372
Difference is 372 – 291 = 81
When I calculate the arithmetic mean of all results for difference, I’ve got that difference between those 2 jars is around 177.
So my final result is: difference is around 180 bonbons.